Redefining Removable Discontinuity : 2 5 4 Extending A Function With A Removable Discontinuity Youtube - Removable discontinuities can be fixed by redefining the function, as shown in the following example.

Redefining Removable Discontinuity : 2 5 4 Extending A Function With A Removable Discontinuity Youtube - Removable discontinuities can be fixed by redefining the function, as shown in the following example.. A removable discontinuity is just something that we can fix or adjust to get the function continuous. Removable discontinuities are also called point discontinuities, because they are small holes in the graph of a function at just a single point. Removable discontinuities can be fixed by redefining the function, as shown in the following example. Formally, a removable discontinuity is one at which the limit of the function exists but does not equal the value of the function at that point; Exists as a finite number.

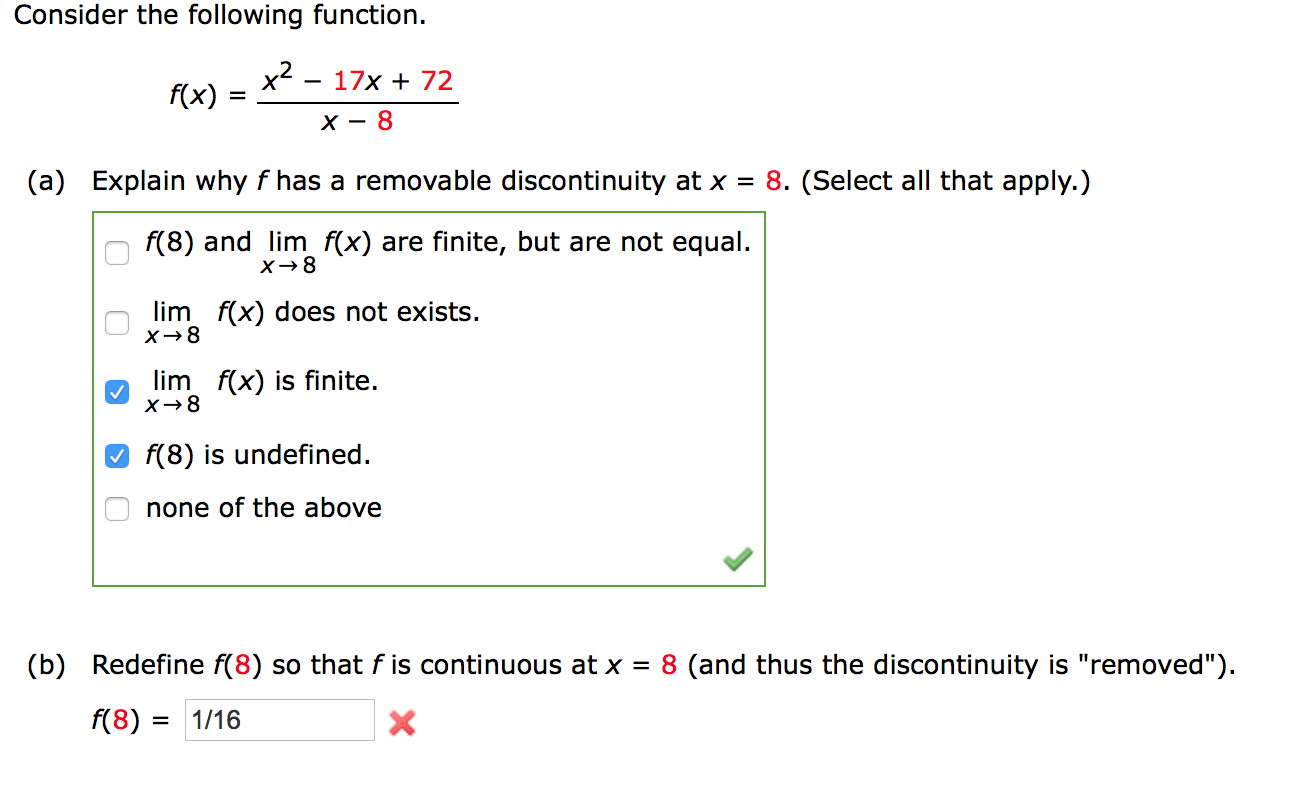
Finally, since (x^2 + 2) > 0 for all x we can conclude that f(x) is continuous for all values. $\begingroup$ that leads it to have a point discontinuity does it not? F(a) could either be defined or redefined so that the new show that f(x) has a removable discontinuity at x=4 and determine what value for f(4) would make f(x) continuous at x=4. When a function has a removable discontinuity, it can be redefined to make it a continuous function. Such discontinuous points are called removable discontinuities.

Removable Discontinuity Myrank from blog.myrank.co.in

This may be because the function does. But we can easily patch a point discontinuity, just by redefining the function at that point. In the example above, to make math processing error continuous you could redefine it as Lim x→a f(x) = f(a). The function below has a removable discontinuity at x = 2. Removable discontinuity a discontinuity is removable at a point x = a if the exists and this limit is finite. Removable discontinuities are removed one of two ways: By redefining the function, we get.

Drag toward the removable discontinuity to find the limit as you approach the hole.

That is, a discontinuity that can be repaired by filling in a single point. A function f(x) is said to have a removable discontinuity at x=a if: Either by defining a blip in the function or by a function that has a common factor or hole in both its denominator and numerator. If you were the one defining the function, you can easily remove the discontinuity by redefining the function. 'removed' the discontinuity and replaced it with an open dot at (2, 1/6). This is the simplest form of discontinuity. It is called removable because this discontinuity can be removed by infinite or essential discontinuity: A removable discontinuity is just something that we can fix or adjust to get the function continuous. F is either not defined or not continuous at x=a. This example leads us to have the following. Removable discontinuity watch more videos at www.tutorialspoint.com/videotutorials/index.htm lecture by: We remove the discontinuity by defining: The first way that a function can fail to be continuous at a point a is that.

This example leads us to have the following. F(a) could either be defined or redefined so that the new show that f(x) has a removable discontinuity at x=4 and determine what value for f(4) would make f(x) continuous at x=4. Of x except x = 2, where it has a removable discontinuity. (a) a function with a nonremovable discontinuity at x = 4 (b) a function with a. A function f(x) is said to have a removable discontinuity at x=a if:

Solved Consider The Following Function F X X2 17x 7 Chegg Com from media.cheggcdn.com

The denominator is 0 for. 2.) the simplest example would be g(x) = 1 / (x + 1). This is the simplest form of discontinuity. Jump and infinite discontinuities are not removable, because we can't easily patch the holes in their graphs. A function f(x) is said to have a removable discontinuity at x=a if: Removable discontinuities are also called point discontinuities, because they are small holes in the graph of a function at just a single point. Finally, since (x^2 + 2) > 0 for all x we can conclude that f(x) is continuous for all values. But f(a) is not defined or f(a) l.

Redefine the function so that in a removable discontinuity, the function can be redefined at a particular point to make it continuous.

Drag toward the removable discontinuity to find the limit as you approach the hole. Notice that for both graphs, even though there are holes at $$x = a$$, the limit value at $$x=a$$ exists. F is either not defined or not continuous at x=a. This example leads us to have the following. In the example above, to make math processing error continuous you could redefine it as Discontinuities for which the limit of f(x) exists and is finite are called removable discontinuities for reasons explained below. The function below has a removable discontinuity at $$x = 2$$. It usually means a function is discontinuous at some point or hole in the graph and all we as long as the limit exists at x= a we could always redefine f(a) to be that limit and remove the discontinuity. That is why it is called a removable type discontinuity. Jump and infinite discontinuities are not removable, because we can't easily patch the holes in their graphs. $\begingroup$ that leads it to have a point discontinuity does it not? A function is said to be discontinuous at a point. This may be because the function does.

A hole in a graph. If we reassign/redefine the function value at can be removed by reassigning the the function value at. But this limit is different from the function value. Removable and nonremovable discontinuities describe the difference between a discontinuity that is removable and a discontinuity that is then give an example of a function that satisfies each description. But f(a) is not defined or f(a) l.

Removable And Jump Discontinuities Differential Calculus Definition Solved Example Problems Exercise Mathematics from img.brainkart.com

F is either not defined or not continuous at x=a. Redefine the function so that it becomes continuous at $$x=2$$. Which we call as, removable discontinuity. But this limit is different from the function value. We remove the discontinuity by defining: Something like removable_discontinuity = true/false. 'removed' the discontinuity and replaced it with an open dot at (2, 1/6). So maybe instead removable_discontinuity=true a better name would be check_limit=true for a potential flag to add to solve().

'removed' the discontinuity and replaced it with an open dot at (2, 1/6).

Removable discontinuities are also called point discontinuities, because they are small holes in the graph of a function at just a single point. F is either not defined or not continuous at x=a. We remove the discontinuity by defining: Removable discontinuities are removed one of two ways: Continuous functions are of utmost importance in mathematics, functions and applications. Removable discontinuity a discontinuity is removable at a point x = a if the exists and this limit is finite. Redefine the function so that it becomes continuous at $$x=2$$. Redefine the function so it is continuous. A removable discontinuity is just something that we can fix or adjust to get the function continuous. Removable discontinuities can be fixed by redefining the function, as shown in the following example. But f(a) is not defined or f(a) l. So if we redefine f of x, if we say f of x is equal to 6x squared plus 18x plus 12 over x squared minus 4. A removable discontinuity is a point on the graph that is undefined or does not fit the rest of the graph.

Cari Blog Ini

elrinconcitodelmanga